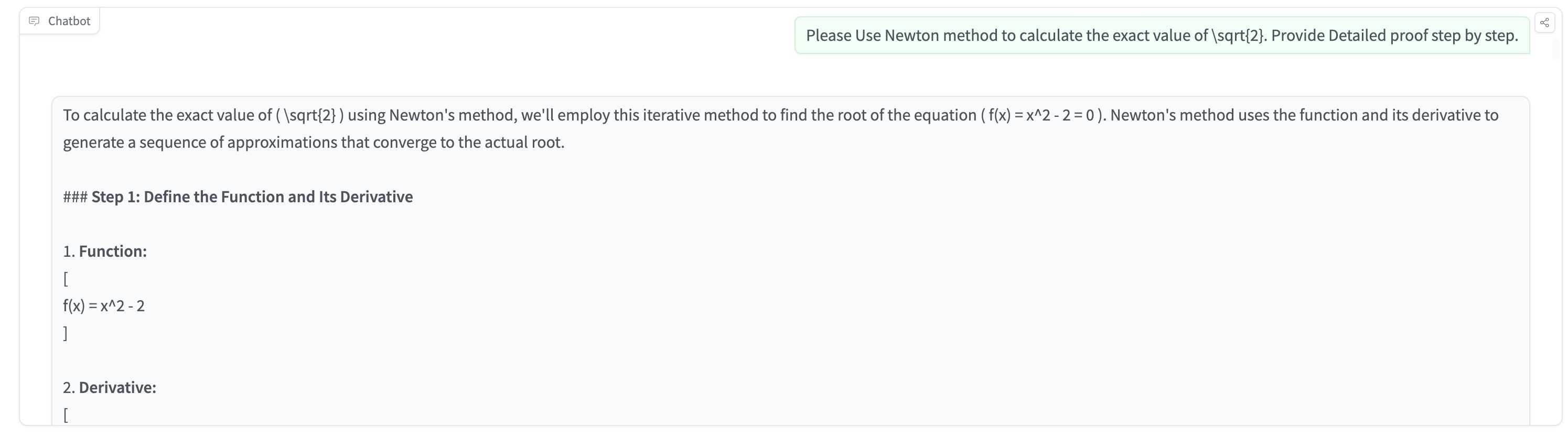
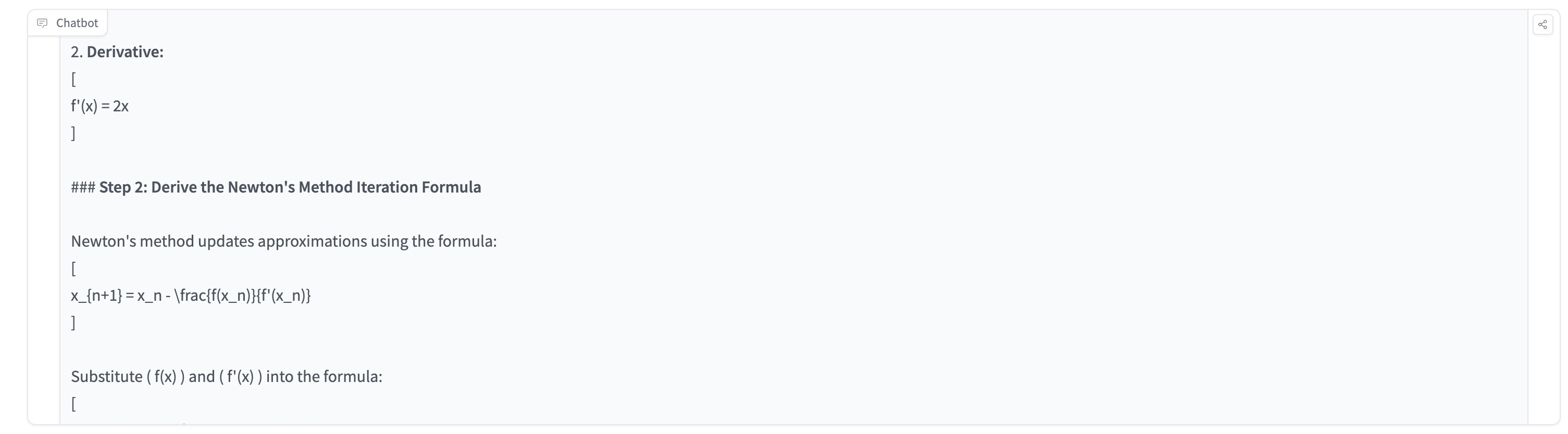
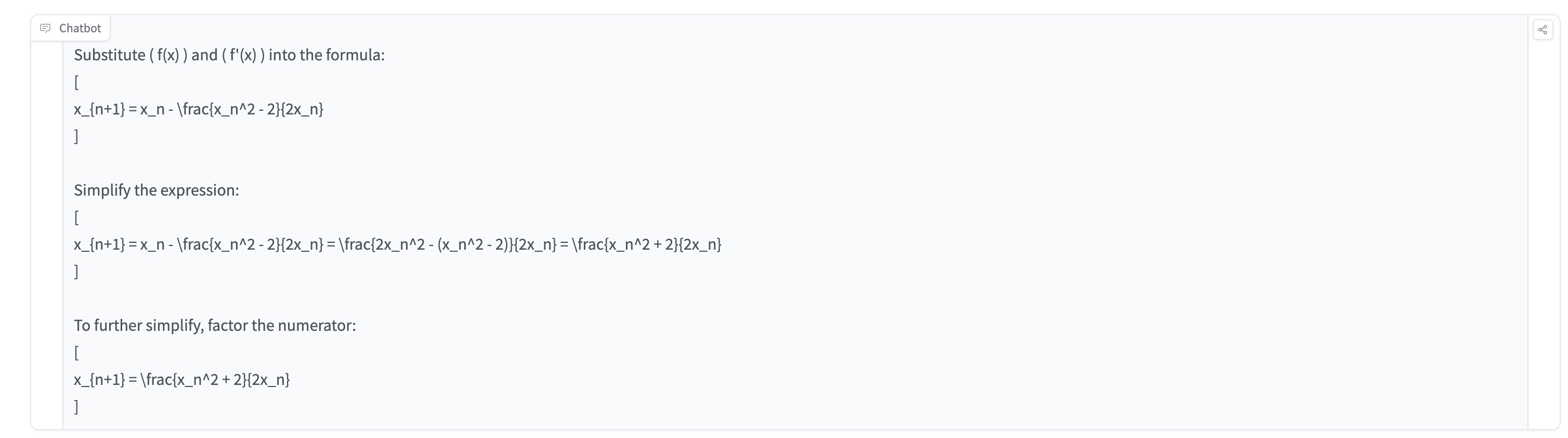
To test OpenAI o1 model's ability of graduate-level machine learning & math, I tried to ask OpenAI o1 to solve the problem of "Use Newton method to calculate the exact value of \sqrt{2}. Provide Detailed proof step by step." OpenAI o1 actually gives me a very long detailed step-by-step proof, which is much detailed and longer than GPT4o or GPT4o-mini. The complete response is very long and I just summarized the detailed steps of the reasoning here. Overall, I think OpenAI o1 can provide more accurate responses than previous GPT4o' answers. But the response usually is very long and there are a lot of top-down break-down, which makes the final result correct but sometimes hard to understand and follow. OpenAI o1 breaks down the machine learning math problem into these steps: ### Step 1: Define the Function and Its Derivative ### Step 2: Derive the Newton's Method Iteration Formula ### Step 3: Choose an Initial Approximation ### Step 4: Perform Iterations ### Step 5: Understanding the Result Each iteration yields a rational approximation that gets closer to ( \sqrt{2} ): - Iteration 1: ( x_1 = \dfrac{3}{2} = 1.5 ) - Iteration 2: ( x_2 = \dfrac{17}{12} \approx 1.4167 ) - Iteration 3: ( x_3 = \dfrac{577}{408} \approx 1.41422 ) - Iteration 4: ( x_4 = \dfrac{667,217}{471,632} \approx 1.41421 ) ### Conclusion Using Newton's method with the function ( f(x) = x^2 - 2 ) and starting approximation ( x_0 = 1 ), we generate a sequence ( { x_n } ) defined by: [ x_{n+1} = \frac{1}{2} \left( x_n + \frac{2}{x_n} \right) ] This sequence converges to ( \sqrt{2} ) as ( n ) approaches infinity. ### Final Answer By applying Newton's method to the equation ( x^2 - 2 = 0 ), we find that ( \sqrt{2} ) is the limit of the sequence: [ x_{n+1} = \dfrac{1}{2} \left( x_n + \dfrac{2}{x_n} \right) ] Starting from ( x_0 = 1 ), each iteration gives a rational approximation of ( \sqrt{2} ). Although we cannot express ( \sqrt{2} ) exactly using Newton's method (since it's irrational), the method provides increasingly accurate approximations.

OpenAI o1 coding ability reviews of reasoning with LLM. In their official website, the prompt for OpenAI o1 is to "takes a matrix represented as a string with format '[1,2],[3,4],[5,6]' and prints the transpose in the same format."After comparing the results of o1 with GPT4o and the final scripts are actually much longer, o1 results have 70 lines of scripts but the GPT4o has only 31 lines of code. The key difference is how to "Build output string". Source: https://openai.com/index/learning-to-reason-with-llms/

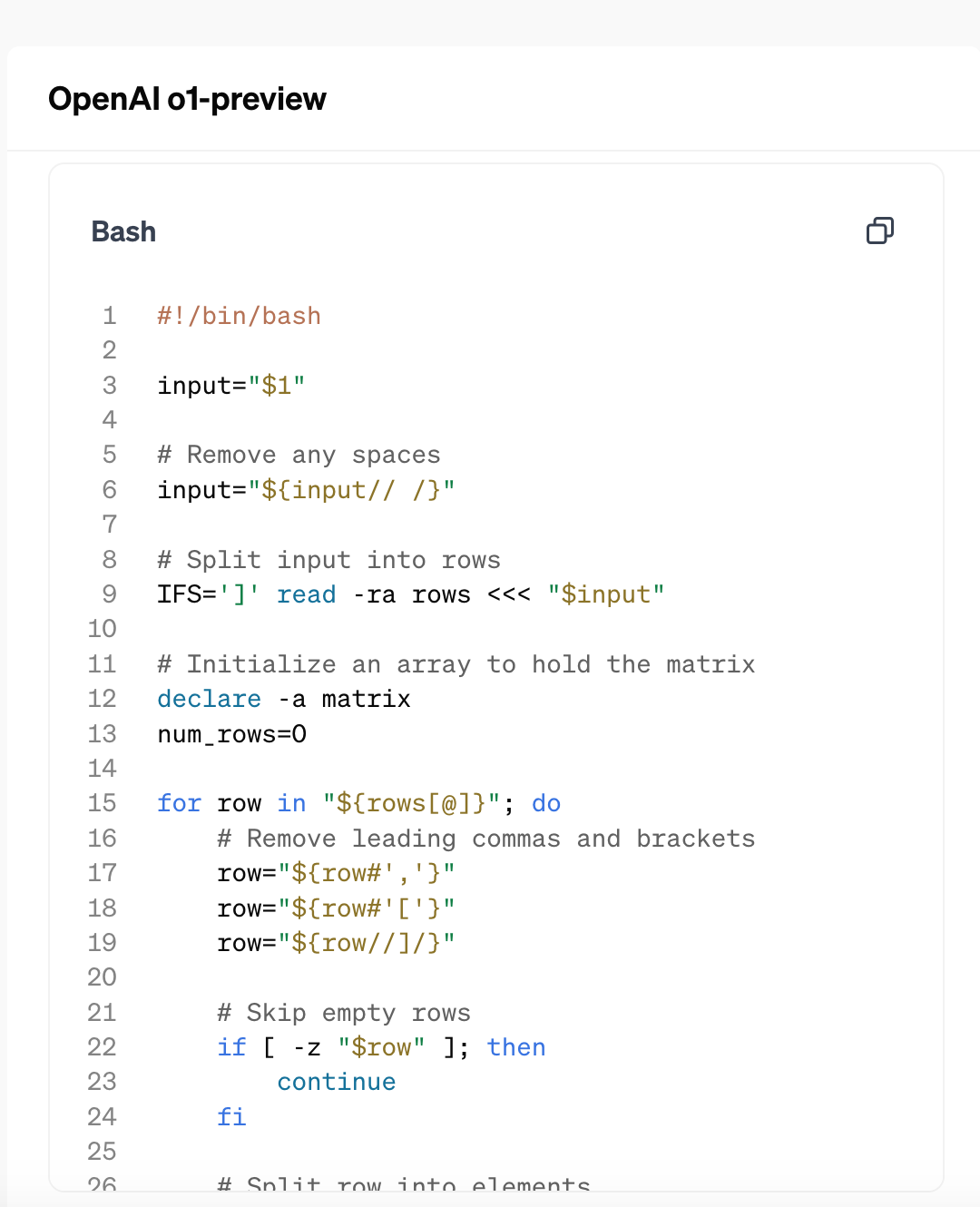
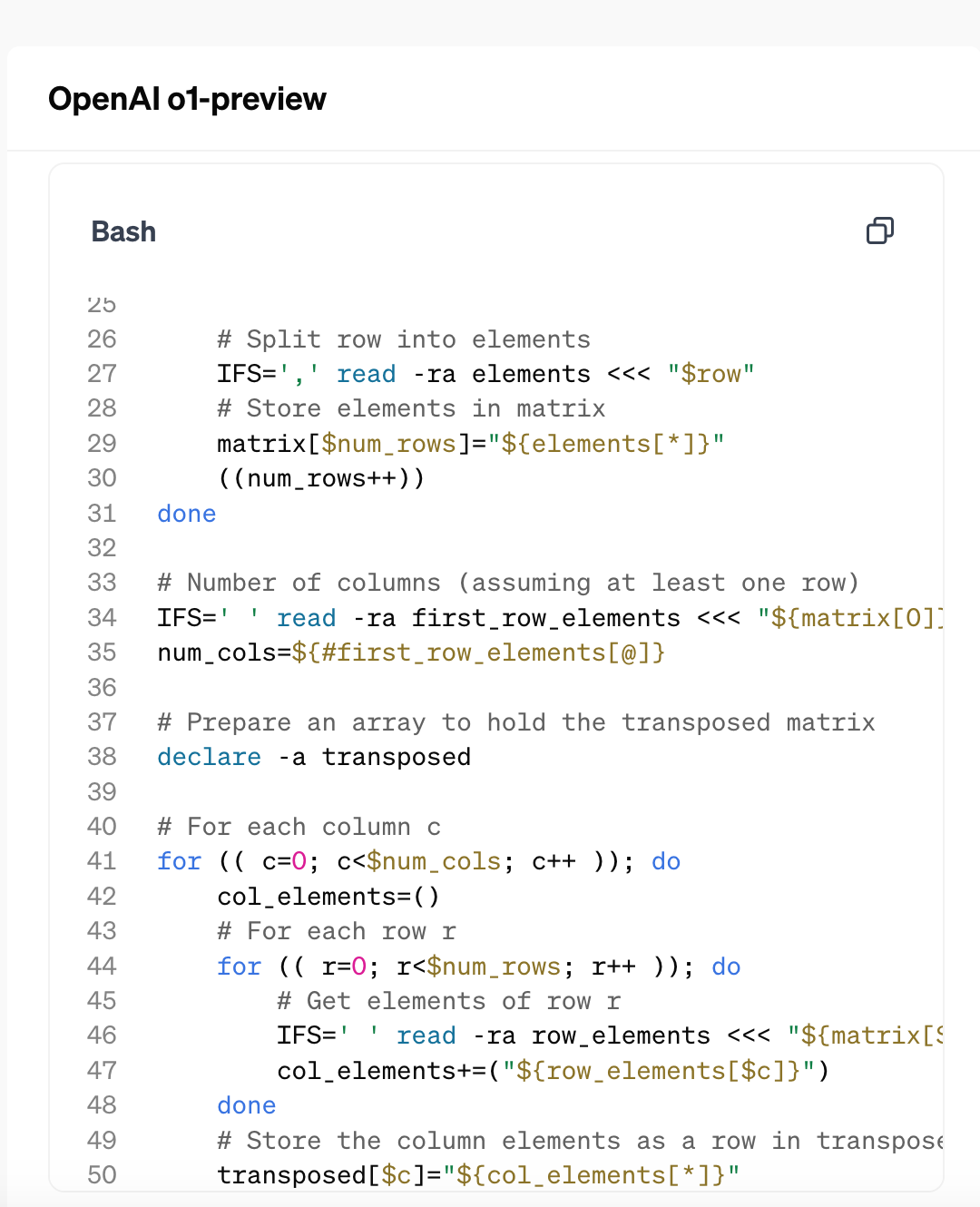
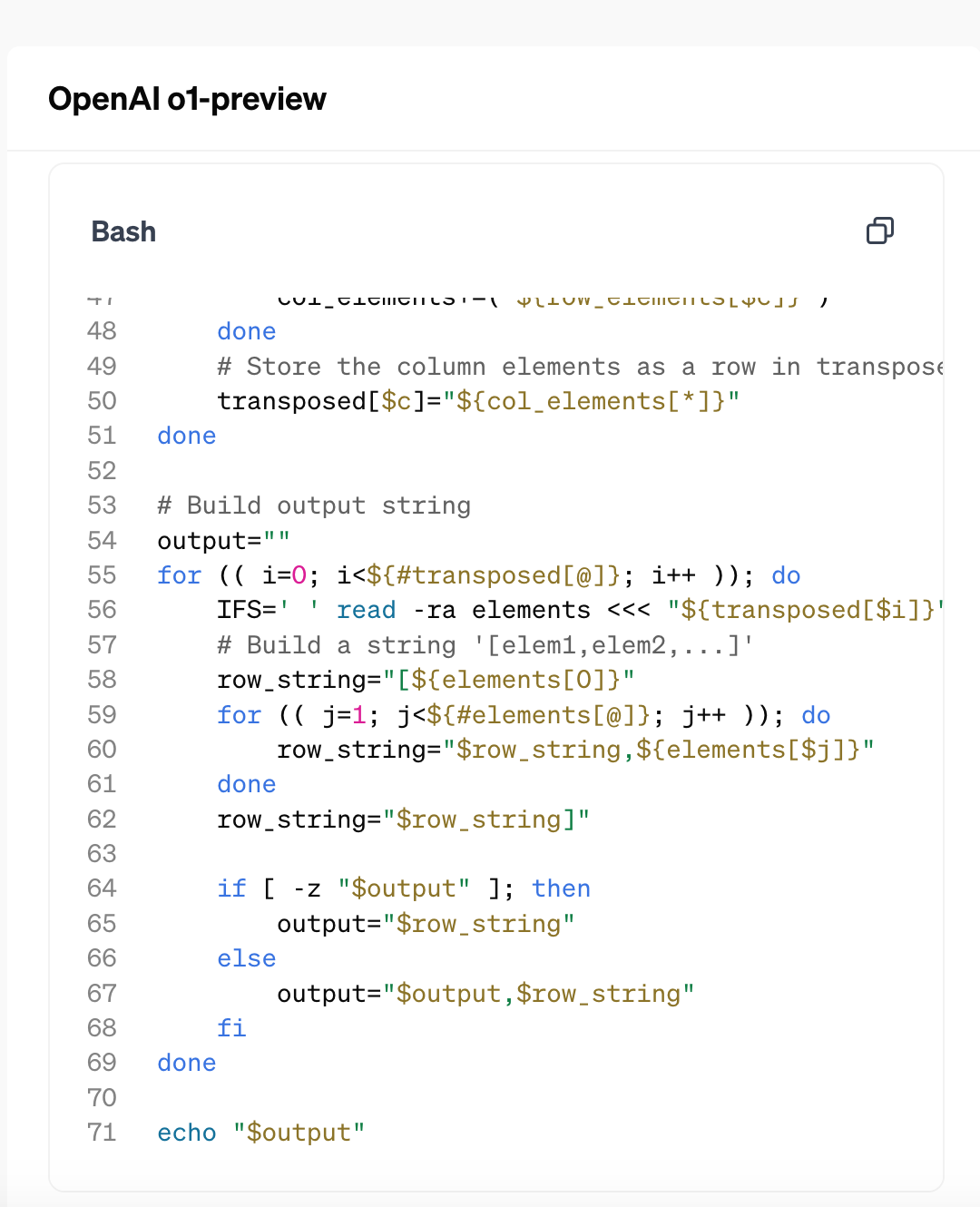
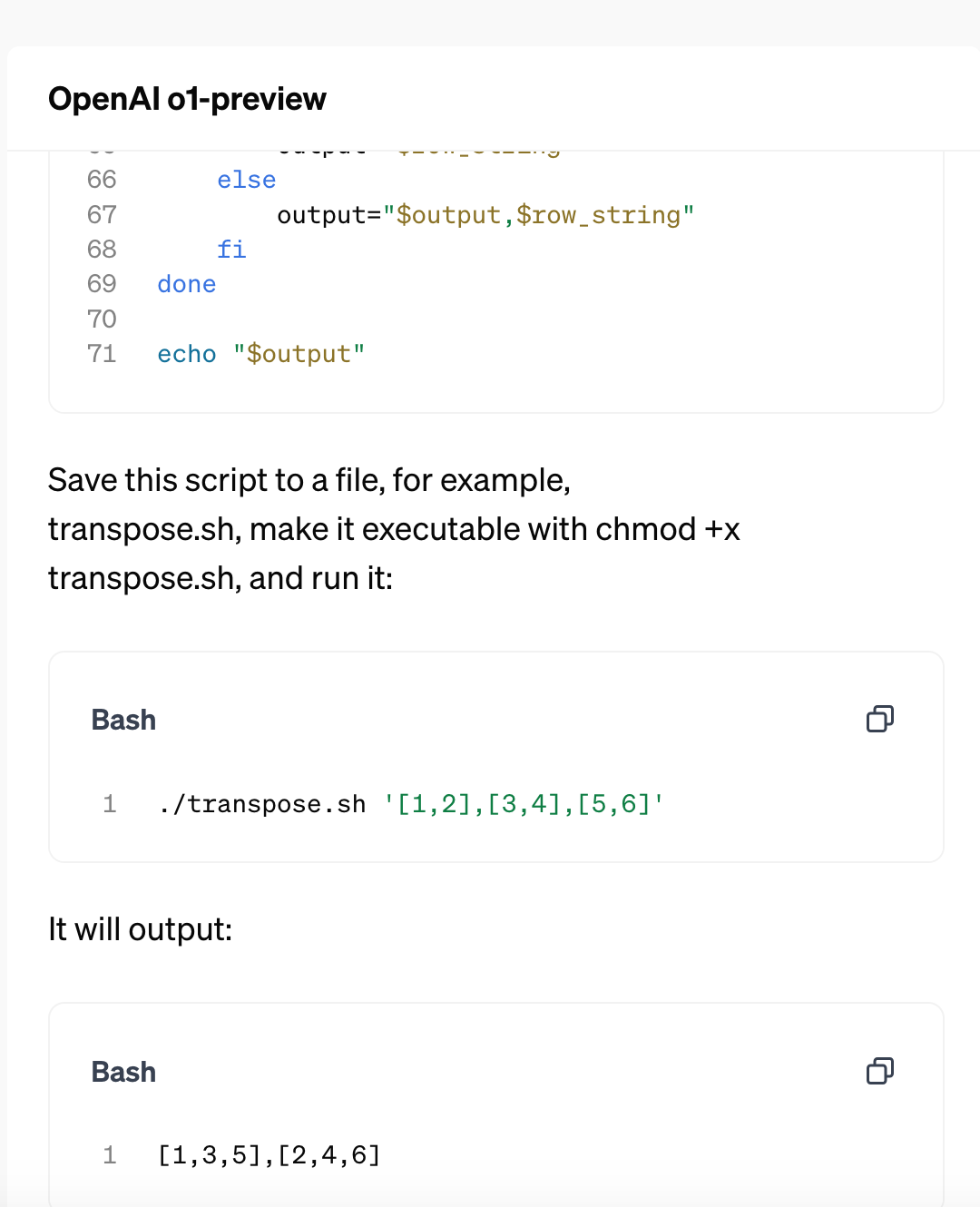
Finally, OpenAI released o1 mdoel with stronger reasoning ability. And I looked through the detailed comparison of a math solving results on their website and the comparison between GPT4o vs OpenAI o1-preview on this Algebra problem. For the math question as the in the prompt, o1 uses a chain of thought when attempting to solve a problem, which is similar to how a human may think for a long time before responding to a difficult question. o1 response actually break down the question into a few steps: "Understanding the Given Information", "Defining a New Polynomial", "Properties of q(x)", "Constructing s(x)", "Matching Coefficients". Finding Additional Solutions and finally reaching Conclusion. The additional real numbers x satisfying p(1/x) = x^{2} are x=\frac{1}{n!} or -\frac{1}{n!}. Overall, the reasoning ability is quite complex compared to previous version, so it's helpful and the answers are correct.